The correct answer is:
The chapter Can You See The Pattern? focuses on dealing with identifying patterns on given sequences. It helps the students to understand how to recognise patterns and point them out in any given situation. It covers the topics: philosophyPatterns in Shapes philosophy Patterns in Numbers philosophyPatterns in alphabets NCERT Math-Magic questions are answered in a simple and engaging manner. We have also related 'Learning Concepts and interactive worksheets with the solutions. Our 'Learning Beyond' segment caters to all the probable questions that a child might think out of curiosity. Download Chapter 7 Can You See The Pattern? in PDF format for free here.
The NCERT Solutions for Maths Class 5 Chapter 7 - Can You See The Pattern? are tailored to help the students master the concepts that are key to success in their classrooms. The solutions given in the PDF are developed by experts and correlate with the CBSE syllabus of 2025-2026. These solutions provide thorough explanations with a step-by-step approach to solving problems. Students can easily get a hold of the subject and learn the basics with a deeper understanding. Additionally, they can practice better, be confident, and perform well in their examinations with the support of this PDF.
Download PDF
Students can access the NCERT Solutions for Maths Class 5 Chapter 7 - Can You See The Pattern?. Curated by experts according to the CBSE syllabus for 2025–2026, these step-by-step solutions make Maths much easier to understand and learn for the students. These solutions can be used in practice by students to attain skills in solving problems, reinforce important learning objectives, and be well-prepared for tests.
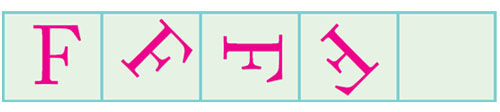
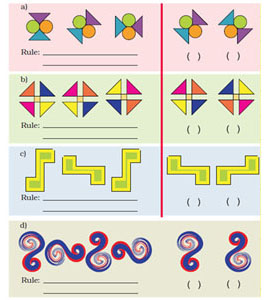
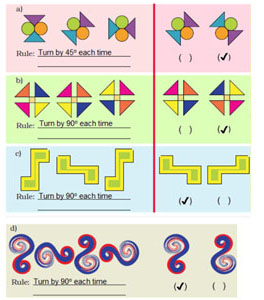
The rule of the pattern is turning by 45° each time. Which will be the next? Tick ( ) the right one.

The correct answer is:

Using the same rule take it forward till you get back to what you started with.


b) Turn each image by 45o to get the next image. The correct answer is:
c) Turn each image by 45o to get the next image. The correct answer is:
What should come next?
a)
b)
c)
d)
a) Observe the pattern in the given shapes. Each shape is one-fourth rotation of the previous shape. The correct answer is: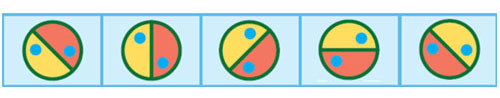
b) Observe the pattern. Each letter is one-fourth rotation of the previous letter. The correct answer is:
c) Observe the pattern in the given shapes. Each shape is one-third rotation of the previous shape. The correct answer is: 
d) Observe the pattern in the given shapes. Each shape is one-fourth rotation of the previous shape. The correct answer is:
Now you use these two rules to make patterns with this block.
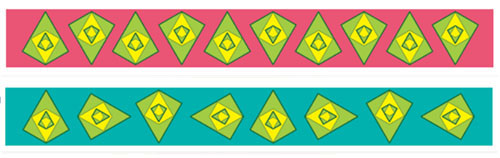
Observe the patterns in the given pictures. In the first picture the shapes are turning by 180o while in the second picture the shapes are turning by 90o
. Therefore, the correct answer is:
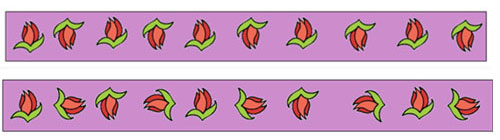
Some patterns are given below on the left side of the red line. For each pattern, write the rule. Then choose what comes next from the right side of the line and tick it.
The correct answer is
Mark that picture which is breaking the rule. Also correct it.
a).
b).
c).
d).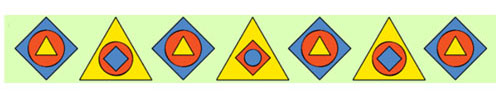
a) Observe the given pattern. Pictures are placed upside and upside down in alternate order. The picture which is breaking the rule is marked below.
Following is the correct pattern image.
b) Observe the given pattern. Every picture is clockwise one-fourth rotation of the previous picture. The picture which is breaking the rule is marked below.
Following is the correct pattern image.
c) Observe the given pattern. Pictures are placed upside and upside down in alternate order. The picture which is breaking the rule is marked below.
Following is the correct pattern image.
d) Observe the given pattern. The first two pictures are placed in an alternate order to make the pattern. The picture which is breaking the rule is marked below.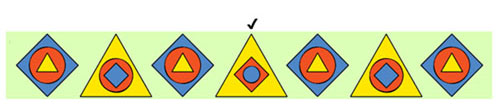
Following is the correct pattern image.
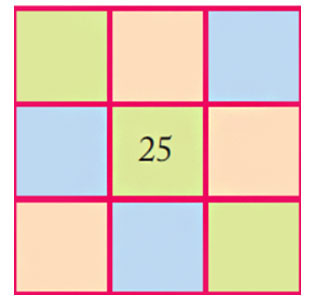
Fill this square using all the numbers from 21 to 29.
Rule: The total of each side is 75.
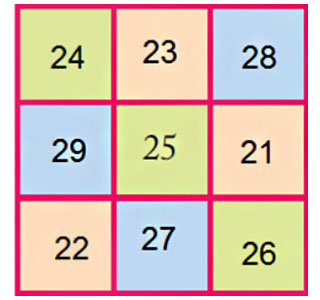
The correct answer is:
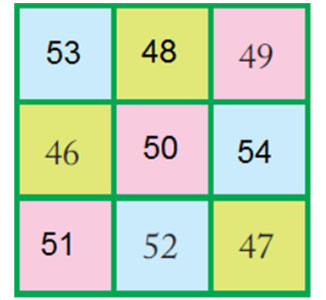
Fill this square using all the numbers from 46 to 54.
Rule: The total of each line is 150.
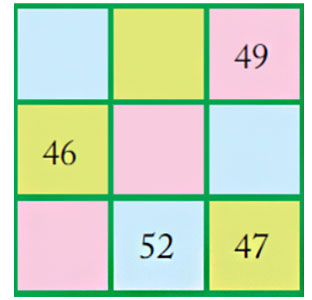
Step 1: Consider the third column.
49 + ___ + 47 = 150
Since 49 + 47 = 96, we have
150 – 96 = 54
Therefore,
49 + 54 + 47 = 150
Hence, write 54 in the empty box of the third column.
Step 2: Now, consider the third row.
__ + 52 + 47 = 150
Since 52 + 47 = 99, we have
150 – 99 = 51
Therefore,
51 + 52 + 47 = 150
Hence, write 51 in the empty box of the third row.
Step 3: Consider the first column, after step 2, we have 51 and 46 in the first column.
51 + 46 + __ = 150
Since 51 + 46 = 97, the missing number is:
150 – 97 = 53
Therefore, write 53 in the empty box of the first column.
Step 4: Consider the first row. Now, we have 53 and 46 in the first row. Therefore,
53 + ___ + 49 = 150.
Since 53 + 49 = 102, the missing number is:
150 – 102 = 48
Therefore, write 48 in the empty box of the first row.
Step 5: Consider the second row. Now, we have 54 and 46 in the second row.
Since 54 + 46 = 100, the missing number is:
150 – 100 = 50
Therefore, write 50 in the empty box of the second row.
The correct answer is:
Take any number. Now multiply it by 2, 3, 4 ............... at every step. Also add 3 to it at each step. Look at the difference in the answer. Is it the same at every step?
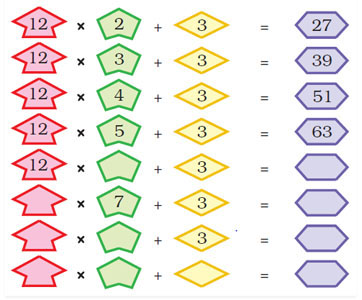
The correct answer is:
Find the difference in answers at each step.
39 – 27 = 12, 51 – 39 = 12, 63 – 51 = 12, 75 – 63 = 12, 87 – 75 = 12, 99 – 87 = 12, and 111 – 99 = 12,
Therefore, the difference in the answers is the same at each step.
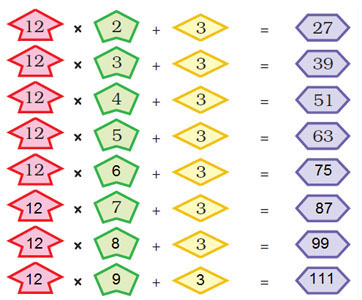
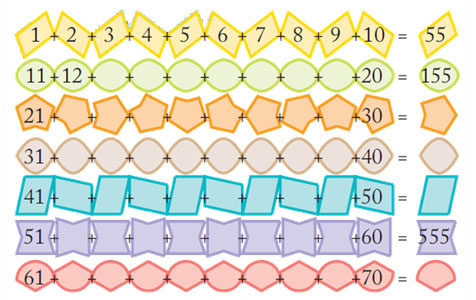
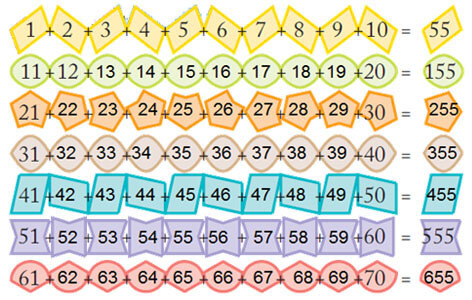
Look at the numbers below. Look for the pattern. Can you take it forward?

The correct answer is

Use the same rule to fill the hexagons below.
a)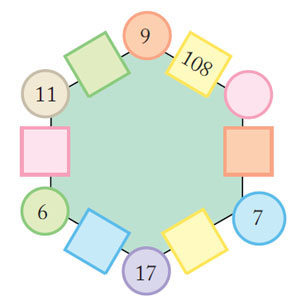
b)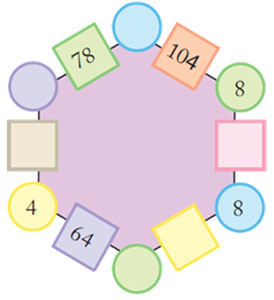
The correct answer is:
a)
b)
Now, look at this-
Check if it is true or not.
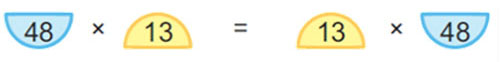
Multiply the given numbers separately.
48 × 13 = 624
13 × 48 = 624
So, the given multiplication statement is true.
Now you try and change these numbers into special numbers a) 28 b) 132 c) 273
a)
Take the number 28.
Turn it backwards: 82.
Add them together: 110.
But this is not a special number because when we reverse its order, we get 011.
Now, add 110 and 011: 121
121 is a special number.
b)
Take the number 132.
Turn it backwards: 231
Add them together: 363
363 is a special number.
c)
Take the number 273.
Turn it backwards: 372
Add them together: 645
But this is not a special number because when we reverse its order, we get 546.
Now, subtract 546 from 645.
645 − 546 = 99
99 is a special number.
Banno and Binod were playing a guessing game by writing clues about a secret number. Each tried to guess the other’s secret number from the clues. Can you guess their secret numbers?
a)
• It is larger than half of 100
• It is more than 6 tens and less than 7 tens
• The tens digit is one more than the ones digit
• Together the digits have a sum of 11
b)
• It is smaller than half of 100
• It is more than 4 tens and less than 5 tens
• The tens digit is two more than the ones digit
• Together the digits have a sum of 6
a)
It is greater than half of 100 that is the number is greater than 50.
It is greater than 6 tens and less than 7 tens that is the number is between 60 and 70.
The ones digit is one less than the tens digit that is the number 65 Therefore, the secret number is 65.
b)
It is less than half of a hundred that is the number is less than 50. It is greater than 4 tens and less than 5 tens that is the number is between 40 and 50.
The ones digit is two less than the tens digit that is the number is 42. Therefore, the secret number is 42.
Fill in the blank spaces in the same way.
a)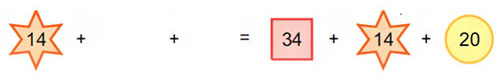
b)
c)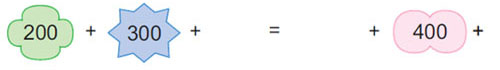
The correct answers are:
a)
b)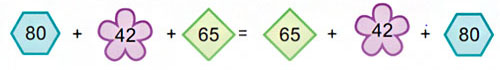
c)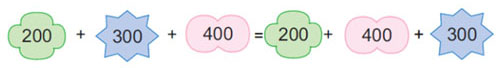
Did you notice some pattern in the answers?
The correct answer is
The difference in the answers is the same at each step that is 100.
Take the first two odd numbers. Now add them, see what you get. Now, at every step, add the next odd number. How far can you go on?
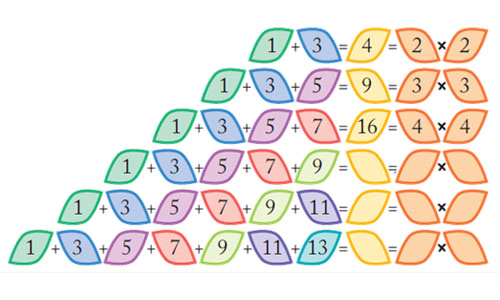
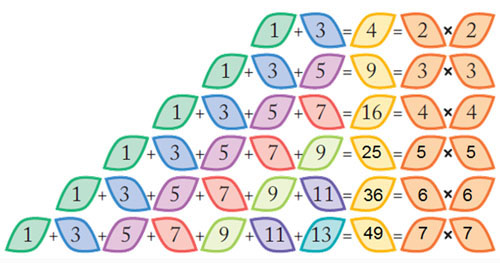
The correct answer is
Since there are countless odd numbers, this pattern will never end.
Are they equal?
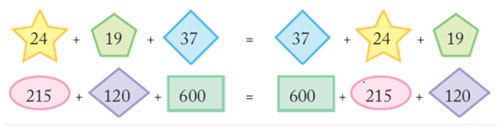
Add the given numbers separately.
24 + 19 + 37 = 80
37 + 24 + 19 = 80
215 + 120 + 600 = 935
600 + 215 + 120 = 935
The change in the order of the numbers in addition does not change the result. So, they are equal.
Look at the patterns of numbers in hexagons. Each side has 2 circles and 1 box.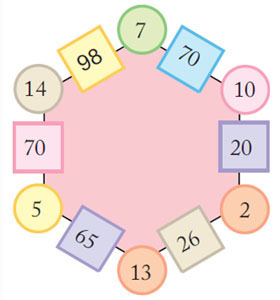
Look at the number 65 in the box. Which are the circles next to it? Can you see how the rule works?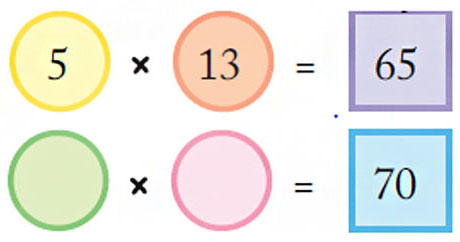
The circles next to 65 are 5 and 13.
The number in each box is the product of the numbers in the circles next to the box.
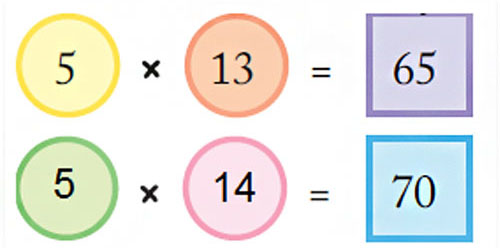
a) Ask your friend — Write down your age. Add 5 to it. Multiply the sum by 2. Subtract 10 from it. Next divide it by 2. What do you get? Is your friend surprised?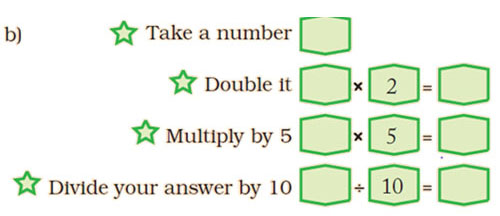
d)Look at this pattern of numbers and take it forward.
a) Do it by yourself. A sample answer is:
Suppose the age of the friend is 11 years.
Add 5 to 11.
5 + 11 = 16
Multiply 16 by 2.
16 × 2 = 32
Subtract 10 from 32.
32 – 10 = 22
Divide 22 by 2.
22 ÷ 2 = 11
The result will always be the same as the age of your friend.
b) Do it by yourself. A sample answer is: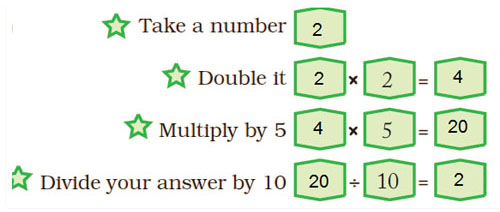
c) Do it by yourself. A sample answer is:
d) The correct answer is:
1 = 1 × 1
121 = 11 × 11
12321 = 111 × 111
1234321 = 1111 × 1111
123454321 = 11111 × 11111
12345654321 = 111111 × 111111
1234567654321 = 1111111 × 1111111
1234567654321 = 1111111 × 1111111
1234567654321 = 1111111 × 1111111
Admissions Open for
Admissions Open for
The NCERT solution for Class 5 Chapter 7: Can You See The Pattern is important as it provides a structured approach to learning, ensuring that students develop a strong understanding of foundational concepts early in their academic journey. By mastering these basics, students can build confidence and readiness for tackling more difficult concepts in their further education.
Yes, the NCERT solution for Class 5 Chapter 7: Can You See The Pattern is quite useful for students in preparing for their exams. The solutions are simple, clear, and concise allowing students to understand them better. They can solve the practice questions and exercises that allow them to get exam-ready in no time.
You can get all the NCERT solutions for Class 5 Maths Chapter 7 from the official website of the Orchids International School. These solutions are tailored by subject matter experts and are very easy to understand.
Yes, students must practice all the questions provided in the NCERT solution for Class 5 Maths Chapter 7: Can You See The Pattern as it will help them gain a comprehensive understanding of the concept, identify their weak areas, and strengthen their preparation.
Students can utilize the NCERT solution for Class 5 Maths Chapter 7 effectively by practicing the solutions regularly. Solve the exercises and practice questions given in the solution.
CBSE Schools In Popular Cities